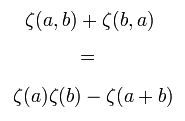Teachers
Karen Yeats works at the interface of combinatorics and quantum field theory. She did her PhD under the physicist Dirk Kreimer. She became an assistant professor at Simon Fraser University in 2009.
Matilde Lalín received her PhD from the University of Texas at Austin. She held a postdoctoral position the Institute for Advanced Study and was a PIMS postdoctoral fellow at University of British Columbia before moving to University of Alberta as an Assistant Professor. She is currently an Associate Professor at the University of Montreal.
Mike Hoffman has been studying multiple zeta values since 1988, when a colleague suggested a problem to him. He is currently Professor of Mathematics at the U. S. Naval Academy, and has held visiting positions at the Max Planck Institute in Bonn in 2003-04 and 2012. He did his doctorate at MIT.
Course
The centrepiece of this program is an intensive course on multiple zeta values and their applications. Over the first two weeks, this will feature 3 hours a day of instruction together with daily problems for students to work on together in the afternoons. Instructors and grad students will be around for the problem sessions.
Multiple zeta values are a class of numbers, believed to be transcendental, which generalize special values of the Riemann zeta function. They relate to many deep topics in modern mathematics, but are also concrete and accessible. We will take a hands on approach to multiple zeta values, playing primarily with discrete objects which underlie them such as Lyndon words, but also looking at some nontrivial consequences in number theory and quantum field theory.
The course is unique opportunity for undergrads to learn an area which is a beautiful link between number theory, combinatorics, and physics, and to reach to research level math using explicit explorations.
Lecture notes
- Notes from Mike Hoffman's lectures.
- Notes (part I), (part II) from Karen Yeats' lectures that weren't really intended for anyone to see. Problems (part I), (part II).
- Notes for July 15 lecture on lattice basis reduction by Nils Bruin.
- Sage computations for using LLL to find integer linear relations between real numbers.
- What was known in 1995.
- A little bit of maple to see for yourself what value K4 gives.
- A few references for what is current
- Schnetz' census: published, arXiv.
- c2 invariants .
- Zigzag conjecture.
- Graphical functions.
- Selected stuff of Panzer.
- The multiple zeta value data mine: published, arXiv.
- Brown's proof of the Hoffman basis and related work:
- Notes from Matilde Lalín's lectures.
Research
During the second two weeks of the program the course load will gradually diminish and students will begin working on group research projects, using Sage to explore the objects we have developed in the course.
Overall this program is suitable for students interested in modern applications of combinatorics to pure mathematics and to physics as well as students interested in number theory and arithmetic geometry.
Credit
This course will be listed for credit at SFU. Students from external universities may be able to obtain course credit as well. Details. Taking the course for credit is not a requirement for participating fully in the program.
Prerequisites
Applicants must have successfully completed basic undergrad calculus, linear algebra, and elementary discrete math (eg MACM 201 at SFU). Ideally a student will have done some upper level work in at least one of those areas. More important is mathematical maturity, a strong interest, and a willingness to get one's hands dirty with mathematical objects.
References
Many of these references are written for research audiences. Program participants are not expected to understand all of these things, but they give some sense of the objects which we will be studying.
- Mike Hoffman's overview of multiple zeta values.
- A course on multiple zeta values.
- A paper which gets into the underlying combinatorics of words.
- Multiple zeta values as they show up in quantum field theory.
- Multiple zeta values as they show up from Mahler measures.